Skip to contents

Function to calculate the area under a selection of information curves

Source: R/areainfo.R
areainfo.Rd

Compute the area of a test or item information function over a definite integral range.

Usage

areainfo(
  x,
  theta_lim,
  which.items = 1:extract.mirt(x, "nitems"),
  group = NULL,
  ...
)

Arguments

x

an object of class 'SingleGroupClass', or an object of class 'MultipleGroupClass' if a suitable group input were supplied

theta_lim

range of integration to be computed

which.items

an integer vector indicating which items to include in the expected information function. Default uses all possible items

group

group argument to pass to extract.group function. Required when the input object is a multiple-group model

...

additional arguments passed to integrate

Value

a data.frame with the lower and upper integration range, the information area within the range (Info), the information area over the range -10 to 10 (Total.Info), proportion of total information given the integration range (Info.Proportion), and the number of items included (nitems)

References

Chalmers, R., P. (2012). mirt: A Multidimensional Item Response Theory Package for the R Environment. Journal of Statistical Software, 48(6), 1-29. doi:10.18637/jss.v048.i06

Author

Phil Chalmers rphilip.chalmers@gmail.com

Examples


dat <- expand.table(LSAT7)
mod <- mirt(dat, 1)
#> 
Iteration: 1, Log-Lik: -2668.786, Max-Change: 0.18243
Iteration: 2, Log-Lik: -2663.691, Max-Change: 0.13637
Iteration: 3, Log-Lik: -2661.454, Max-Change: 0.10231
Iteration: 4, Log-Lik: -2659.430, Max-Change: 0.04181
Iteration: 5, Log-Lik: -2659.241, Max-Change: 0.03417
Iteration: 6, Log-Lik: -2659.113, Max-Change: 0.02911
Iteration: 7, Log-Lik: -2658.812, Max-Change: 0.00456
Iteration: 8, Log-Lik: -2658.809, Max-Change: 0.00363
Iteration: 9, Log-Lik: -2658.808, Max-Change: 0.00273
Iteration: 10, Log-Lik: -2658.806, Max-Change: 0.00144
Iteration: 11, Log-Lik: -2658.806, Max-Change: 0.00118
Iteration: 12, Log-Lik: -2658.806, Max-Change: 0.00101
Iteration: 13, Log-Lik: -2658.805, Max-Change: 0.00042
Iteration: 14, Log-Lik: -2658.805, Max-Change: 0.00025
Iteration: 15, Log-Lik: -2658.805, Max-Change: 0.00026
Iteration: 16, Log-Lik: -2658.805, Max-Change: 0.00023
Iteration: 17, Log-Lik: -2658.805, Max-Change: 0.00023
Iteration: 18, Log-Lik: -2658.805, Max-Change: 0.00021
Iteration: 19, Log-Lik: -2658.805, Max-Change: 0.00019
Iteration: 20, Log-Lik: -2658.805, Max-Change: 0.00017
Iteration: 21, Log-Lik: -2658.805, Max-Change: 0.00017
Iteration: 22, Log-Lik: -2658.805, Max-Change: 0.00015
Iteration: 23, Log-Lik: -2658.805, Max-Change: 0.00015
Iteration: 24, Log-Lik: -2658.805, Max-Change: 0.00013
Iteration: 25, Log-Lik: -2658.805, Max-Change: 0.00013
Iteration: 26, Log-Lik: -2658.805, Max-Change: 0.00011
Iteration: 27, Log-Lik: -2658.805, Max-Change: 0.00011
Iteration: 28, Log-Lik: -2658.805, Max-Change: 0.00010

areainfo(mod, c(-2,0), which.items = 1) #item 1
#>  LowerBound UpperBound      Info TotalInfo Proportion nitems
#>          -2          0 0.3899825 0.9879254  0.3947489      1
if (FALSE) { # \dontrun{
areainfo(mod, c(-2,0), which.items = 1:3) #items 1 to 3
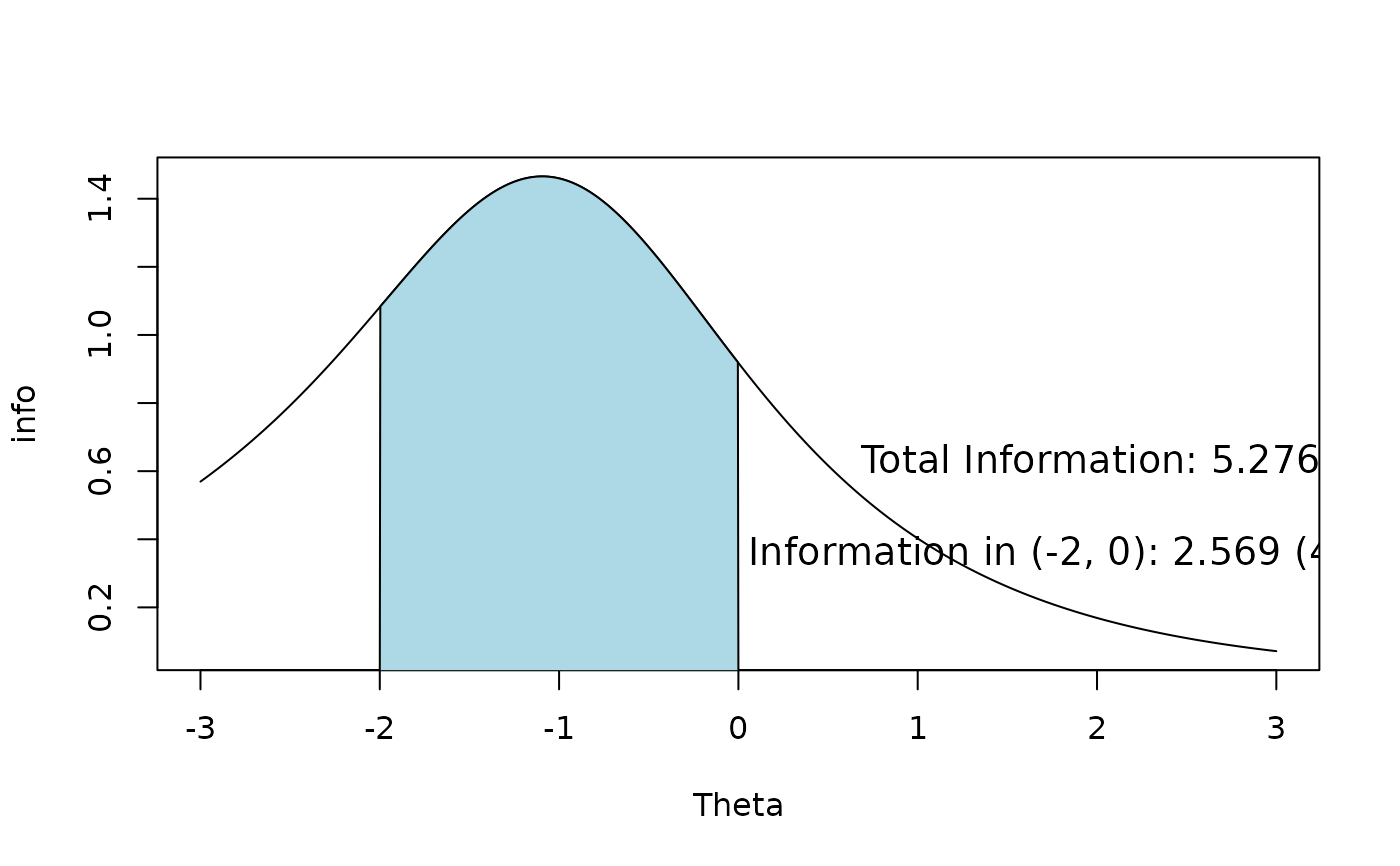
areainfo(mod, c(-2,0)) # all items (total test information)

# plot the area
area <- areainfo(mod, c(-2,0))
Theta <- matrix(seq(-3,3, length.out=1000))
info <- testinfo(mod, Theta)
plot(info ~ Theta, type = 'l')

pick <- Theta >= -2 & Theta <=0
polygon(c(-2, Theta[pick], 0), c(0, info[pick], 0), col='lightblue')
text(x = 2, y = 0.5, labels = paste("Total Information:", round(area$TotalInfo, 3),
           "\n\nInformation in (-2, 0):", round(area$Info, 3),
           paste("(", round(100 * area$Proportion, 2), "%)", sep = "")), cex = 1.2)

} # }